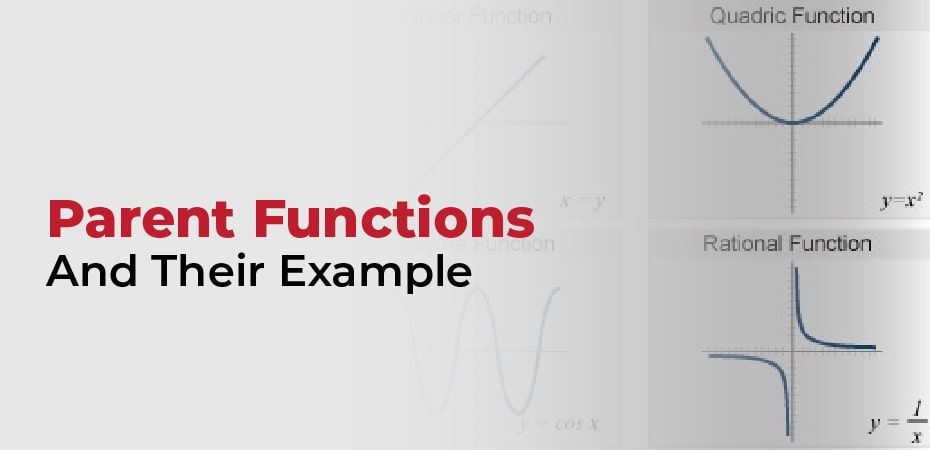
A parent function is when two functions are very close to each other and create a new function out of them. Exactly how similar does it get? In the same way that we and our family members share similar characteristics, genes, and behaviors, families of functions share similar algebraic properties, have similar graphs, and behave in similar ways.
As an example of a family of functions, consider the quadratic functions. There are certain characteristics common to all quadratic functions, such as their graphs are parabolas, and their graphs all have the same shape. However, we can find similarities between our own parents’ and parents’ functions. Families of functions are groups of related functions in mathematics. Just as we have parents in our own families, there are parents in families of functions.
Worksheet For Parent Functions
The Greatest Integer Function, also known as the Step Function, is a function that returns the most significant integer between a number and its next highest equivalent (similar to rounding down to the nearest integer). There is also a function called the Least Integer Function, which is indicated by //(y=/left/floor x //right/ceil), which returns the least integer greater or equal to a number (imagine rounding one up to an integer).
We assume that the “parent functions” pass through the origin (/left( [0,0] /right)/), or if it doesn’t pass through the origin, it isn’t shifted at all.
To figure out what end behavior a function has, we simply look at the smallest and largest values of /(X)’s and ask which direction the /(y) is going. Functions that go back and forth with their /(y/) values and never really go way up or way down (called periodic functions), don’t have an end behavior.
Last of the time, our end actions looks being like this ( displaystyle begin battery l x to- infty handbook, y to? x to infty handbook,y to? end battery) and we’ve to fill in the (y) part. So the end actions for a line with a positive cant is ( begin battery l x to- infty handbook, y to- infty x to infty handbook, y to infty end battery).
We do not have the negative portion of the /(x/) end behavior when the start of the /(x/) is 0 (such as in the radical function). The so-called positive (right) side of x is equally indicated by the /displaystyle x/to [[0]* [+]/text., y/to -/infty/). This is illustrated in the log function.
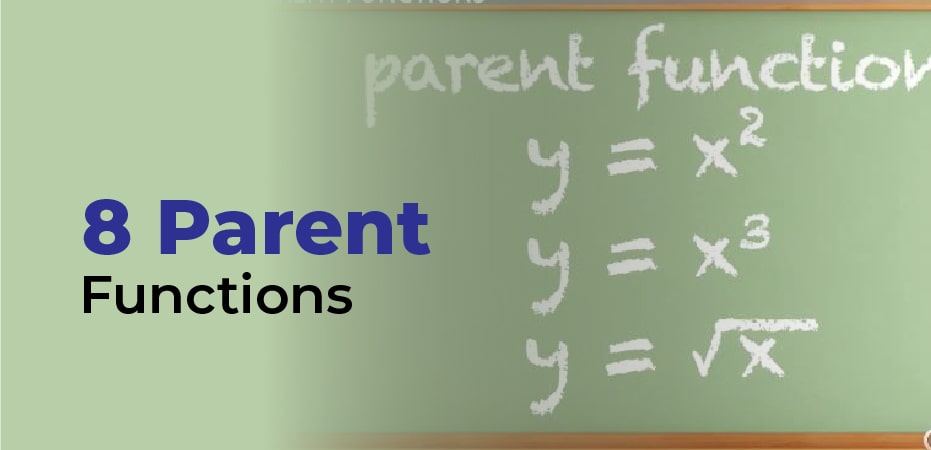
8 Parent Functions
- It is f(x)=e that defines the exponential function.
- Linear function f(x)=x.
- There are 8 parents.
- A Logistic Function of 1 is f(x). f(x+1) = 1.
- This suggests bounding.
- Cubing functions are f(x) = x.
- F(x) is equal to cos x.
Is There A Definition Of A Parent Function?
Among the different types of functions, you can find are quadratic functions. It is defined as the simplest function that still meets the definition of a certain function type. As an example, there would be a parent function for each linear function in a family of linear functions.
Graphs Of The Parent Functions
Include basic linear, quadratic, cubic, rational, absolute value, and square root parent functions.
Compare graphs with equations. Make matches between family names and functions. Analyze graphs by family names. Identify cards accurately by reading them carefully.
Matching exercises are designed for this activity. This will not work as a flashcard exercise.
In the table below you will find the rules for transforming functions. You can see examples and solutions for using the transformation rules at the bottom of this page.
[Image here]
Chart Of Parent Functions
With the help of T-charts, we can make use of function transformations. For instance, on the off chance that you realize that the quadratic parent work \(y={{x}^{2}}\) is being changed 2 units to one side, and 1 unit down (just a shift, not a stretch or a flip yet), we can make the first t-graph, following by the change focuses outwardly of the first focuses. To obtain the newly transformed function, plot the “outside” points.
In the examples that follow, you move the function up and down and perform “regular” math when functions are transformed outside of an (f(x)/) part. This transformation or translation affects the y-axis of the function.
Transforming the (f(x)) part inside of an equation is the opposite of moving the x (you would move everything to the other side if you isolated just x). These are horizontal transformations or translations of a function’s /(x/) part.
T-charts work consistently across all functions, which is great for transforming parent functions. It should be noted that I included more than just the critical points above in most t-charts, just to make the graphs more interesting.
Formulas & Worksheets For Parent Functions & Transformations
According to the previous paragraph, there is a parent function for every family of functions. No simpler function still fits the definition of their type than a parent function. A linear family of functions would be defined as y = x when they are thought of as linear functions. Essentially, this is the most basic linear function.
The graph of a parent function can be transformed to produce all the functions within a family of functions. Horizontal shifts, vertical or horizontal stretching and compression, reflection over x or y axes, and vertical shifts are all examples of these transformations. Y = 2x*2 + 4x, in the above graph, represents the parent function y = x*2 shifted one unit left, stretched vertically, and shifted down two units. Since no general shape is changed, all functions in a family look like their parent functions.
As a result of these transformations, the parent function is added to or subtracted from, or multiplied by a constant. It is possible to deduce the function y = 2x*2 + 4x by taking its parent function y = x*2 and multiplying it by the constant 2 before adding 4x to it.