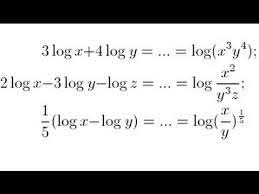If you’ve ever wondered how to write multiple logarithms as a single logarithm, you’re in the right place! In this article, we’ll explore the definition of logarithms, their basic properties, and the change of base formula.
We’ll also cover combining logarithms with the same and different bases, solving equations with single logarithms, and real-world applications.
By the end, you’ll have a clear understanding of what can be written as a single logarithm. Let’s dive in!
Definition of Logarithms
Do you understand what logarithms are and how they’re defined? Well, let me break it down for you.
Logarithms are mathematical tools that help us solve problems involving exponential growth or decay. They’re the inverse operation of exponentiation.
In simpler terms, logarithms allow us to find the exponent to which a certain base must be raised to obtain a given number. For example, if we’ve the equation 10 raised to the power of x equals 100, the logarithm base 10 of 100 is equal to 2.
Logarithms have various properties that make them useful in solving equations, such as the power rule and the product rule. Understanding logarithms is essential in a wide range of fields, including mathematics, science, and engineering.
Basic Properties of Logarithms
You should remember that the basic properties of logarithms, such as the power rule and the product rule, are essential to understanding their usefulness in solving equations.
By applying these properties, you can simplify complex logarithmic expressions and solve equations more efficiently.
The power rule states that when you have a logarithm raised to a power, you can bring the power down to the front as a coefficient. For example, if you have log base 2 of x squared, you can rewrite it as 2 times log base 2 of x.
Similarly, the product rule allows you to split a logarithm of a product into the sum of logarithms of the individual factors. This rule is particularly helpful when dealing with equations containing multiple terms or variables.
The Change of Base Formula
The Change of Base Formula allows you to simplify logarithms by converting them to a different base. By using this formula, you can rewrite logarithms in a way that makes them easier to work with.
Applying the Change of Base Formula is a useful skill that can help you solve logarithmic problems more efficiently.
Simplifying Logarithms With Formula
Use the formula to simplify logarithms and combine them into a single expression.
When faced with multiple logarithms, it can be helpful to simplify and combine them to make the expression more manageable.
The formula you can use is log base a of b multiplied by log base a of c equals log base a of b times c. This allows you to combine logarithms with the same base into a single logarithm.
For example, if you have log base 2 of x plus log base 2 of y, you can simplify it to log base 2 of xy.
This simplification can make equations easier to solve and calculations more efficient.
Applying Formula to Problems
When solving logarithm problems, remember to apply the formula for the change of base to simplify and combine logarithms. This formula allows you to rewrite logarithms in a different base, making them easier to work with.
To apply the formula, first identify the base of the logarithm you want to change. Then, divide the logarithm by the logarithm of the new base. This will give you the new logarithm in the desired base.
Finally, simplify and combine any logarithms if possible. By using the formula for the change of base, you can transform complex logarithms into simpler forms, which will help you solve logarithm problems more efficiently.
Combining Logarithms With the Same Base
When combining logarithms with the same base, you can simplify the expression by adding the logarithmic terms together.
For example, if you have log(base a) of x plus log(base a) of y, you can combine them as log(base a) of (xy).
This simplification allows you to condense the expression into a single logarithm.
Simplifying Logarithmic Expressions
You can simplify logarithmic expressions by combining logarithms with the same base. When you have multiple logarithms with the same base, you can use the properties of logarithms to simplify the expression into a single logarithm.
To do this, you need to understand the properties of logarithms, such as the product rule, quotient rule, and power rule.
The product rule states that when you have the logarithm of a product, you can separate it into the sum of the logarithms of each factor.
The quotient rule states that when you have the logarithm of a quotient, you can separate it into the difference of the logarithms of the numerator and denominator.
The power rule states that when you have the logarithm of a power, you can bring the exponent down and multiply it with the logarithm.
Adding Logarithmic Terms
To simplify logarithmic expressions, add logarithmic terms with the same base into a single logarithm. This process allows you to condense multiple logarithmic terms into one, making the expression easier to work with.
Let’s say you have two logarithmic terms with the base 2: log2(x) + log2(y). By adding these terms together, you can combine them into a single logarithm: log2(xy). This simplification reduces the complexity of the expression and makes it more manageable for further calculations.
Keep in mind that this technique only applies when the logarithmic terms have the same base. If the bases are different, you can’t combine the terms into one logarithm.
Remember to use this method to simplify logarithmic expressions and make your mathematical work more efficient.
Combining Logarithms With Different Bases
There’s no need to worry about combining logarithms with different bases; you can simplify the expression to a single logarithm.
When you have logarithms with different bases, you can use the change of base formula to rewrite them in terms of a common base. This formula allows you to convert any logarithm to a base 10 or base e logarithm.
Once you have rewritten the logarithms with the same base, you can combine them by using the properties of logarithms. The most commonly used property is the logarithmic identity, which states that log base b of a multiplied by log base a of b is equal to 1.
By applying this property, you can simplify the expression and evaluate it to a single logarithm with the desired base.
Solving Equations With Single Logarithms
There are several strategies you can use to solve equations with single logarithms.
One common method is to use the properties of logarithms to rewrite the equation in a simpler form. For example, if you have an equation with a logarithm on both sides, you can use the property of logarithmic equality to combine them into a single logarithm.
Another strategy is to use exponentiation to eliminate the logarithm. You can rewrite the equation using exponential form and solve for the variable.
Additionally, if the equation has a logarithm with a known base, you can use the power rule to simplify the equation and solve for the variable.
It’s important to remember to check your solutions as logarithmic equations can sometimes have extraneous solutions.
Applications of Single Logarithms
You can apply single logarithms to simplify complex expressions and solve exponential equations. By combining multiple logarithms into a single logarithm, you can condense the expression and make it easier to work with. This is particularly useful when dealing with large numbers or complicated calculations.
Single logarithms can also help solve exponential equations by converting them into logarithmic form. This allows you to isolate the variable and find its value.
In addition, single logarithms can be used to convert between different bases. By using the properties of logarithms, you can rewrite the expression in terms of a different base, which can be more convenient for calculations or comparisons.
Overall, understanding how to apply single logarithms can greatly simplify mathematical problems and make them more manageable.
Conclusion
So, now you understand the concept of single logarithms. They’re a powerful tool in simplifying and solving equations. By combining logarithms with the same base or using the change of base formula, you can condense multiple logarithms into a single one. This not only simplifies calculations but also helps in solving various mathematical problems.
Single logarithms have wide applications in fields like finance, science, and engineering. So, embrace the power of single logarithms and unlock new possibilities in your mathematical journey.