What Does Rhombus Mean in Geometry

Have you ever wondered what a rhombus is? The term may sound new to some people, but it has widespread geometry use. The definition of a rhombus is an important building block for other geometric concepts.

A rhomb is any four-sided figure with opposite sides parallel to each other and all sides of equal length. Though the shapes of the lines that make up the figure can be varied from straight to curved, they will always have bilateral symmetry since only two rotations are needed for it to look exactly like its original composition.

Read on to learn more about this powerful geometrical object!

What is Rhombus?

Rhombus = Rhomb

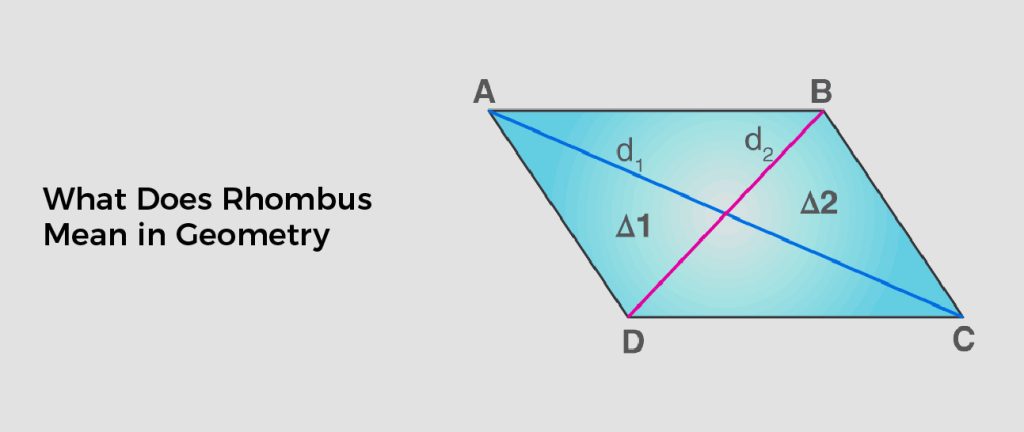
A rhombus is a parallelogram of four equal sides and a special type of quadrilateral. They have two pairs of opposite sides that are parallel and perpendicular. Moreover, each rhomb has a pair of perpendicular diagonals that bisect each other.

The length of the two diagonals is equal to the side length. Moreover, the area of a rhombus is half the product of the length of each diagonal.

One feature of a rhomb is that the angle between two diagonals is 90 degrees. Moreover, rhombuses have two axes of symmetry. Here is the formula for types of rhombus: the area of the rhombus and the perimeter of a rhombus.

• Area of a Rhombus = (Length of Diagonal 1 x Length of Diagonal 2)/2
• The perimeter of a Rhombus = 4 x Side length.

How is a Rhomb Different from a Square?

A square has all right angles, while a rhomb does not need to have all right angles. Therefore, a square is formed by a rhomb with right angles.

A rhombus is not necessarily a triangular pyramid. It could be a square, hexagon, or octagon. The standard metric of length is usually around 16 feet, 7 inches. Some bridges may have variable message signs in lieu of traditional traffic signage. A more streamlined message might be in order if it’s a well-known junction involving multiple lanes.

One of the best places to see a rhombus in action is at a local amusement park. Most rides have a ride-on program to keep visitors safe from harm, and it’s a good idea to keep a few eyeballs on the prize. Besides, a little extra time to enjoy the park is better than the alternative. Likewise, splurging on a new car or a nice dinner at a fancy restaurant is not harmful if you’ve got a little extra cash in your pocket.

The rhombus is a good example of a talisman. It is a polygon shaped like the pubic zone. In Russian, it is called a romb. But the more esoteric lozenge has replaced it in the modern world.

Properties of a Rhombus

• All sides of the rhombus shape are equal, like AB = BC = CD = DA.
• Diagonals bisect each other at 90 degrees, like AC and BD bisect each other at 90 degrees.
• Opposite sides are parallel in a rhomb like AB || and AD || BC.
• Opposite angles are equal in a rhomb shape, like ∠A = ∠C and ∠B = ∠D.
• Adjacent angles add up to 180°.
• The interior angles of the rhomb can add up to 360 degrees.
• Adjacent angles of a rhomb add up to 180 degrees.
• The diagonals of a rhomb bisect each other at right angles and form four congruent isosceles triangles.
• The diagonals of a rhomb bisect each other like DI = BI and AI = CI.
• A rhombus has rotational symmetry of 180° (order 2). That is, a rhombus retains its original orientation when rotated by an angle of 180°.
• The diagonals of a rhomb are the only two lines of symmetry. The rhomb is divided into two identical halves by these lines.

Example of Rhombus

• EXAMPLE NO 1:

The length of the two diagonals of a rhombus is 12cm and 8cm. Find the area of the rhomb.

Solution:

Diagonal (d1) = 12cm

Diagonal (d2) = 8cm

Area of rhombus = (d1×d2)2 = (12×8)2 sq.cm = 48 sq.cm

• EXAMPLE NO 2:

Find the perimeter of the rhomb with its side measuring 35cm.

Solution:

Length of the side of the rhomb (a) = 35cm

Perimeter of rhombus = 4 × a = 4 × 35cm = 140cm

• EXAMPLE NO 3:

The length of the two diagonals of a rhomb is 25cm and 6cm. Find the area of the rhombus.

Solution:

Diagonal (d1) = 25cm

Diagonal (d2) = 6cm

Area of rhomb = (d1×d2)2 = (25×6)2 sq.cm = 75 sq.cm