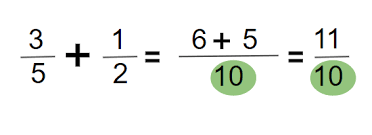Adding fractions with different denominators can sometimes present a bit of a challenge, requiring a methodical approach to ensure accurate results. You may find yourself pondering how to seamlessly combine these fractions, but fear not, as we have a clear and concise step-by-step guide to assist you in navigating this mathematical terrain.
Each step is designed to lead you towards a solution that is both precise and easily comprehensible. So, let’s embark on this journey together and unlock the secrets to mastering fraction addition with varying denominators.
Understanding Fraction Denominators
Do you know why understanding fraction denominators is crucial for adding fractions with different denominators?
The denominator of a fraction represents the total number of equal parts into which a whole is divided. When adding fractions with different denominators, it’s essential to comprehend these denominators to ensure accurate calculations.
If the denominators of the fractions you’re adding are different, you must first find a common denominator before proceeding with the addition. The denominator determines the size of each part of the whole, so having a clear grasp of this concept is fundamental. Without a common denominator, the fractions can’t be added together directly.
Understanding fraction denominators allows you to determine how many parts of the whole each fraction represents. This knowledge enables you to adjust the fractions so they have the same denominator, making it possible to combine them accurately. By mastering the concept of fraction denominators, you pave the way for successfully adding fractions with different denominators.
Finding a Common Denominator
To find a common denominator when adding fractions with different denominators, you need to identify the least common multiple of the denominators. The least common multiple is the smallest number that both denominators can divide into evenly.
Start by listing the multiples of each denominator. For example, if you have fractions with denominators 4 and 6, the multiples of 4 are 4, 8, 12, 16, 20, and so on, while the multiples of 6 are 6, 12, 18, 24, 30, and so forth.
Next, look for the smallest number that appears in both lists. In this case, 12 is the least common multiple of 4 and 6.
Once you have identified the least common multiple, you can use it as the common denominator for your fractions. By converting the fractions to have this common denominator, you can then add or subtract them easily. Remember, finding a common denominator is crucial for performing operations with fractions efficiently.
Converting Fractions to Like Denominators
When adding fractions with different denominators, ensuring they’ve like denominators by converting them is essential for seamless arithmetic operations. To convert fractions to like denominators, you first identify the least common denominator (LCD) by finding the least common multiple (LCM) of the denominators. Once you have the LCD, you rewrite each fraction with the LCD as the new denominator while adjusting the numerators accordingly.
For example, consider adding 1/3 and 1/4. The denominators are 3 and 4, and the LCM is 12. To convert 1/3 to have a denominator of 12, you multiply both the numerator and denominator by 4, resulting in 4/12. Similarly, to convert 1/4 to have a denominator of 12, you multiply both the numerator and denominator by 3, yielding 3/12. Now, both fractions have a denominator of 12, making it easy to add them together. Converting fractions to like denominators simplifies the addition process and allows for accurate calculations.
Adding Numerators of Like Denominators
To calculate the sum of numerators with like denominators, simply combine the numerators directly. When adding fractions with the same denominator, you only need to focus on adding the numerators together.
For example, if you have 1/5 + 2/5, since the denominators are the same, which is 5 in this case, you can directly add the numerators to get 3/5. This straightforward process makes adding fractions with like denominators much simpler compared to when the denominators are different.
By adding the numerators directly, you’re essentially consolidating the parts of the fractions that represent the quantity being added. Remember, the denominator remains the same in the resulting fraction, and you only need to add or subtract the numerators based on the operation you’re performing.
Mastering this step is crucial before moving on to simplifying the resulting fraction in the next stage of adding fractions with different denominators.
Simplifying the Resulting Fraction
After adding numerators of like denominators, the next step is simplifying the resulting fraction to its lowest terms.
Simplifying a fraction involves reducing it to the smallest possible whole numbers. To simplify a fraction, look for the greatest common factor (GCF) between the numerator and the denominator. Divide both the numerator and the denominator by their GCF until the fraction can no longer be reduced further.
For example, if you have the fraction 6/12, you can simplify it by finding the GCF of 6 and 12, which is 6. Dividing both the numerator and the denominator by 6 gives you 1/2, the simplest form of the fraction.
Practice Problems and Examples
You can enhance your understanding of adding fractions with different denominators by engaging in practice problems and examples. By working through various scenarios where the fractions have distinct denominators, you can solidify your grasp on the concept and improve your computational skills.
For example, consider adding 1/4 + 2/3. To solve this, you need to find a common denominator, which in this case would be 12. Then, convert 1/4 and 2/3 into fractions with a denominator of 12: 1/4 becomes 3/12, and 2/3 becomes 8/12. Add these fractions together to get 11/12 as the result.
Another practice problem could involve adding 3/5 + 1/6. Find the common denominator, which is 30 in this case. Convert 3/5 to 18/30 and 1/6 to 5/30. Add these fractions to get 23/30.
Frequently Asked Questions
Yes, you can add fractions with different denominators without finding a common denominator using other methods like converting the fractions to equivalent fractions with the same denominator. It simplifies the process.
To know if a fraction is in its simplest form before adding it to another, check if the numerator and denominator have no common factors other than 1. Simplify by dividing both by the greatest common factor.
To quickly add fractions with different denominators, find the least common denominator (LCD) by multiplying the denominators together. Then, convert each fraction to have the LCD. Finally, add the numerators together and simplify if needed.
Conclusion
Now that you know how to add fractions with different denominators, you can confidently tackle any fraction problem that comes your way.
Remember to find a common denominator, convert fractions to like denominators, add the numerators, and simplify the result.
With practice and patience, you’ll be a fraction addition pro in no time!
Keep up the good work and keep learning.