‘Slow and steady wins the race.’
In the vast world of geometry, trapezoids can sometimes be a tricky shape to navigate. But fear not, for this how-to guide will equip you with the knowledge and skills to confidently find the midsegment of a trapezoid.
Whether you’re a student looking to ace your geometry exam or simply someone curious about the wonders of mathematics, understanding midsegments in trapezoids is a valuable skill to possess.
So, let’s embark on this geometric journey together, unraveling the secrets and properties of midsegments, and discovering the formula that will unlock the hidden treasures of trapezoid midsegments.
Get ready to expand your mathematical horizons and embark on a captivating adventure into the world of trapezoids.
What Is a Trapezoid?
A trapezoid is a four-sided polygon with at least one pair of parallel sides. It’s a shape that you may encounter in geometry problems or in real-life situations. The defining feature of a trapezoid is its parallel sides, which are called bases. The other two sides are called legs. The bases can be of different lengths, but they must be parallel to each other. The legs can also have different lengths, and they connect the bases at non-parallel angles.
When you look at a trapezoid, you can notice that it doesn’t have any right angles. The angles formed between the legs and the bases can vary. However, the sum of the measures of the two non-parallel angles is always equal to 180 degrees. This property of trapezoids can be useful when solving problems involving angles.
Trapezoids can come in different shapes and sizes. Some trapezoids have equal legs, making them isosceles trapezoids. Other trapezoids may have one of the legs longer than the other, making them scalene trapezoids. Understanding the basic properties of a trapezoid is essential when working with more complex concepts involving this shape.
Understanding Midsegments in Geometry
To understand midsegments in geometry, let’s start by defining what they are.
Midsegments are line segments that connect the midpoints of two sides of a triangle or a trapezoid. They’ve some important properties, such as being parallel to the third side of the triangle or trapezoid and being half the length of that side.
Additionally, midsegments can be constructed by connecting the midpoints of the sides using a straight line.
Definition of Midsegments
Understanding midsegments in geometry is crucial for comprehending the concept of finding the midsegment of a trapezoid.
So, what exactly is a midsegment? Well, in geometry, a midsegment is a line segment that connects the midpoints of two sides of a triangle or a quadrilateral.
In the case of a trapezoid, the midsegment is the line segment that connects the midpoints of the two non-parallel sides. The midsegment is always parallel to the two bases of the trapezoid and is equal to half the sum of the lengths of the two bases.
This definition is important because it provides the foundation for finding the midsegment of a trapezoid, which can be useful in various geometric calculations and proofs.
Properties of Midsegments
One important property to understand about midsegments in geometry is that they’re always parallel to the two bases of a trapezoid. This means that if you were to draw a line segment connecting the midpoints of the two non-parallel sides of a trapezoid, that line segment would be parallel to the two bases.
This property holds true for all trapezoids, regardless of their shape or size. It’s an important concept to grasp, as it allows us to make certain conclusions about the relationships between the sides and angles of a trapezoid.
For example, if we know that the midsegment is parallel to the bases, we can use this information to find missing angles or lengths within the trapezoid. Understanding the parallel nature of midsegments is crucial when working with trapezoids in geometry.
Construction of Midsegments
Constructing midsegments in geometry is a fundamental skill that allows us to analyze and understand the properties of trapezoids. To construct a midsegment of a trapezoid, start by drawing the two non-parallel sides of the trapezoid. Then, connect the midpoints of these two sides with a straight line segment. This line segment is the midsegment of the trapezoid. It’s parallel to the bases and is located halfway between them.
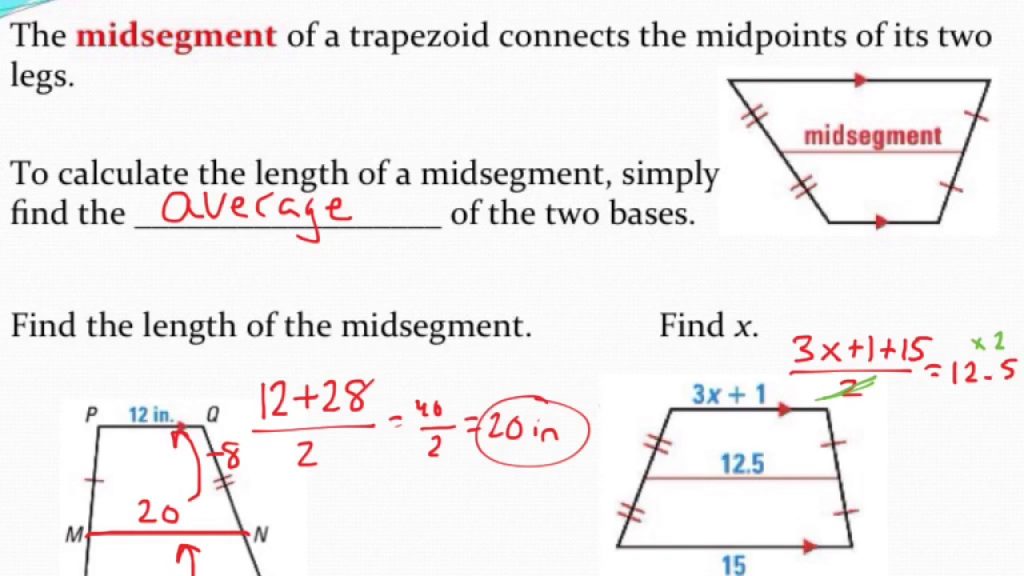
The length of the midsegment can be found by taking the average of the lengths of the two bases. By constructing the midsegment, we gain insights into the relationships between the sides and angles of the trapezoid. This construction is a valuable tool in studying and analyzing trapezoids.
The Definition of a Midsegment in a Trapezoid
When finding the midsegment of a trapezoid, it’s helpful to define the midsegment as the line segment that connects the midpoints of the two non-parallel sides. In simpler terms, it’s the line that runs parallel to the trapezoid’s bases and is located halfway between them. To understand this definition better, let’s break it down.
First, a trapezoid is a quadrilateral with exactly one pair of parallel sides. The parallel sides are known as the bases, and the other two sides are called the legs. The midsegment, as defined earlier, connects the midpoints of the legs.
To find the midsegment, you need to locate the midpoints of the two legs. The midpoint of a line segment is the point that divides it into two equal halves. Once you have found the midpoints, draw a line segment connecting them. This line segment is the midsegment.
The midsegment of a trapezoid has some interesting properties. It’s always parallel to the bases and is equal in length to the average of the bases. This means that if you know the lengths of the bases, you can easily find the length of the midsegment by taking their average.
Understanding the definition of a midsegment is crucial when solving problems involving trapezoids. It serves as a foundation for further calculations and analysis of trapezoid properties.
Properties of Midsegments in Trapezoids
The properties of midsegments in trapezoids reveal important relationships between the midsegment, bases, and legs of the trapezoid. Understanding these properties can help you solve problems involving trapezoids and their midsegments.
One important property is that the midsegment of a trapezoid is parallel to the bases. This means that the midsegment lies in the same plane as the bases, and its length is equal to the average of the lengths of the bases. For example, if the lengths of the bases are 6 and 10 units, then the length of the midsegment would be (6 + 10) / 2 = 8 units.
Another property is that the midsegment of a trapezoid is also equal in length to the sum of the lengths of the legs. This means that if you know the lengths of the legs, you can find the length of the midsegment by adding them together.
Furthermore, the midsegment divides the trapezoid into two smaller trapezoids, each of which has the same height as the original trapezoid. This property can be useful when finding the area of a trapezoid using the formula:
Area = (1/2) * (b1 + b2) * h,
where b1 and b2 are the lengths of the bases and h is the height.
The Formula for Finding the Midsegment of a Trapezoid
To find the midsegment of a trapezoid, simply divide the sum of the lengths of the bases by 2. The formula for finding the midsegment of a trapezoid is straightforward and easy to apply.
Suppose you have a trapezoid with bases of lengths b1 and b2. To find the midsegment, you first add the lengths of the bases together: b1 + b2. Once you have the sum, you divide it by 2 to get the midsegment length. Mathematically, the formula is expressed as (b1 + b2) / 2 = midsegment length.
Let’s take an example to illustrate this formula. Suppose you have a trapezoid with a shorter base, b1, measuring 6 units and a longer base, b2, measuring 10 units. By applying the formula, you add the lengths of the bases: 6 + 10 = 16. Then, you divide the sum by 2: 16 / 2 = 8.
Therefore, the midsegment length of this trapezoid would be 8 units.
Step-by-Step Guide: Finding the Midsegment of a Trapezoid
To find the midsegment of a trapezoid, start by adding the lengths of its bases together, and then divide the sum by 2. This step-by-step guide will help you find the midsegment with ease.
- Identify the lengths of the bases: The trapezoid has two bases – the longer one and the shorter one. Let’s call them base 1 and base 2.
- Add the lengths of the bases: Take the length of base 1 and add it to the length of base 2. This will give you the total sum of the bases.
- Divide the sum by 2: Take the total sum of the bases and divide it by 2. This will give you the midsegment length.
- Label the midsegment: Once you have found the midsegment length, label it as midsegment.
Example Problem: Finding the Midsegment of a Trapezoid
Now let’s apply the step-by-step guide to solve an example problem on finding the midsegment of a trapezoid.
Imagine you have a trapezoid with a parallel side length of 10 units and a non-parallel side length of 6 units. To find the midsegment, you need to follow a few simple steps.
First, find the average of the two parallel sides. In this case, add the lengths of the parallel sides (10 + 6) and divide by 2. The average is 8 units.
Next, draw a line segment connecting the midpoints of the non-parallel sides. This line segment is the midsegment. In our example, draw a line connecting the midpoint of the side with length 10 to the midpoint of the side with length 6.
Common Mistakes to Avoid When Finding the Midsegment
Avoid these common mistakes when finding the midsegment of a trapezoid to ensure accurate results.
One common mistake is forgetting to identify the bases of the trapezoid correctly. Remember that the bases are the parallel sides of the trapezoid, and they’re the ones used to calculate the midsegment.
Another mistake to avoid is using the wrong formula. The formula for finding the midsegment of a trapezoid is the average of the lengths of the two bases. Some people mistakenly use the formula for finding the midsegment of a triangle, which is incorrect.
Additionally, be careful not to confuse the midsegment with the height of the trapezoid. The midsegment is a line segment that connects the midpoints of the two non-parallel sides of the trapezoid, while the height is perpendicular to the bases.
Lastly, make sure to measure the lengths of the bases accurately. A small measurement error can lead to significant discrepancies in the calculated midsegment length.
Applications of Midsegments in Real Life
Midsegments of trapezoids have various practical applications in real life situations. One of the most common applications is in the construction industry. When building a roof, for example, the midsegment of a trapezoid can help determine the length of the ridge beam needed to support the roof structure. By finding the midsegment, construction workers can accurately calculate the dimensions required for a secure and stable roof.
Another application of midsegments can be found in transportation and urban planning. When designing roads and highways, engineers use midsegments to determine the optimal length of exit and entrance ramps. By finding the midsegment of the trapezoidal-shaped interchange, engineers can ensure smooth traffic flow and safe merging.
Midsegments also play a role in architecture and interior design. When designing rooms or buildings with irregular shapes, such as trapezoidal windows or doors, midsegments can help determine the correct dimensions for a visually pleasing and functional design.
In the field of manufacturing, midsegments are used in the design and production of various products. For example, in the automotive industry, midsegments are utilized to calculate the dimensions of car parts, ensuring that they fit together correctly and function properly.
Further Resources and Practice Problems
To further explore the topic of midsegments and strengthen your understanding, here are some additional resources and practice problems that can help you solidify your knowledge.
If you’re looking for more in-depth explanations and examples, online tutorials and videos can be a great resource. Websites like Khan Academy and MathisFun offer comprehensive lessons on geometry topics, including midsegments. These tutorials break down the concept step-by-step and provide clear explanations, making it easier for you to grasp the material.
In addition to online resources, practice problems are crucial for reinforcing your understanding of midsegments. You can find plenty of practice worksheets and exercises in geometry textbooks or on educational websites. These problems will challenge you to apply the concepts you’ve learned and help you develop problem-solving skills.
Another way to practice is by working through sample test questions. Many standardized tests, like the SAT or ACT, include geometry questions that involve midsegments. By familiarizing yourself with these types of questions, you’ll be better prepared for any assessments you may encounter.
Frequently Asked Questions
Can a Trapezoid Have More Than One Midsegment?
Yes, a trapezoid can have more than one midsegment. These midsegments are segments that connect the midpoints of the legs of the trapezoid.
How Is the Midsegment of a Trapezoid Different From the Midsegment of a Triangle?
The midsegment of a trapezoid is different from the midsegment of a triangle because it connects the midpoints of the non-parallel sides, whereas the midsegment of a triangle connects the midpoints of any two sides.
What Is the Relationship Between the Midsegment and the Bases of a Trapezoid?
The midsegment of a trapezoid is parallel to the bases and is half the sum of their lengths. It connects the midpoints of the legs and is a helpful tool in finding the area of the trapezoid.
Are There Any Special Properties of Isosceles Trapezoids Related to the Midsegment?
In isosceles trapezoids, the midsegment is parallel to the bases and its length is equal to the average of the lengths of the bases. This special property helps in solving problems involving isosceles trapezoids.
Can the Midsegment of a Trapezoid Be Longer Than Any of Its Bases?
No, the midsegment of a trapezoid cannot be longer than any of its bases. It is always shorter than both bases because it connects the midpoints of the non-parallel sides.
Conclusion
In conclusion, understanding midsegments in trapezoids is essential in geometry. By knowing the definition, properties, and formula for finding the midsegment, you can solve problems with ease.
Avoid common mistakes and apply this knowledge to real-life situations. Remember to utilize further resources and practice problems to strengthen your understanding.
Geometry becomes more manageable when you grasp the concept of midsegments in trapezoids.