Dimensional Analysis – Principle, Applications and Example

Where the Dimensional Analysis is used? Matching and switching between various units is a very useful and important skill. We perform this each day without obtaining it. For example, when we follow a procedure, we may require to do easy conversions, similar to switching grams to ounces, or quarts to cups.

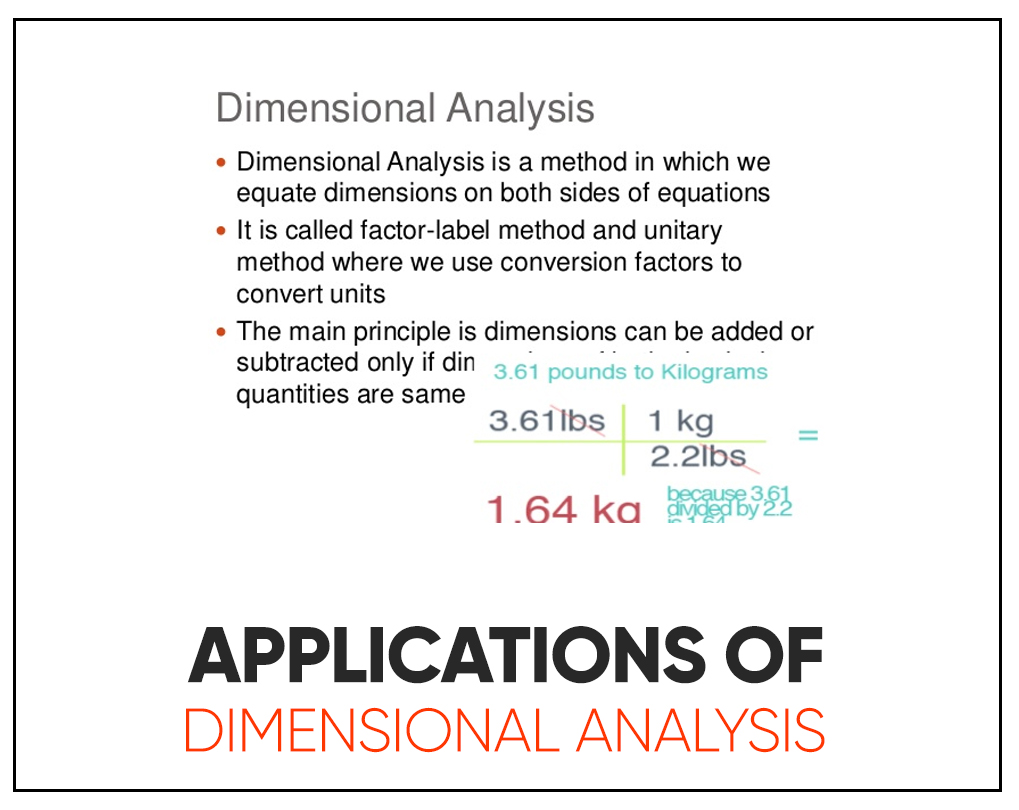
In math and science, we often change a number or amount with a dimensional unit to a separate unit, similar meters to kilometers. Dimensional analysis, likewise known as the unit-factor method or factor-label method, is a way to switch 1 different type of unit to another. By using this way, we can transform into a separate unit, but their values are equal. To transform one unit to another, we perform the use of a conversion factor, which is a numerical amount that we can multiply or divide to the quantity or number that we need to convert.

For example, if I want to know how many yards are there in 10 feet, we can recall that 3 feet are equivalent to 1 yard. Then, I can use the method of dimensional analysis and transform feet into yards by applying the conversion factor shown below in yellow. If I need to understand how many minutes there are in 2 hours, I can apply the conversion factor.

Dimensional analysis is something that we have accomplished without knowing it. We transform minutes to hours, or days to hours the whole time. Also, if we move to a different country that usually equates distance by kilometers rather of miles, then we switch between the 2 units as well by practicing the dimensional analysis approach.

Dimensional Analysis is also called Factor Label Method or Unit Factor Method as we use conversion factors to get the same units. As an instance, if we need to know the total minutes in three hours, what we will do? We commonly evaluate as follows:

60 minutes <=1 hour

180 minutes <= 3 × 60 minutes <= 3 hour

(Here the conversion factor 60 minutes)

We practice conversion factors respectively so that the solution comes in the aspired unit and biased outcomes are avoided.

Applications of Dimensional Analysis 

This Analysis is a very essential aspect of measurement and has many applications in the real world. We use this method for 3 prominent reasons, they are:

• A fixture of a dimensional equation
• Determine the relationship among physical quantities in physical phenomena
• To convert units from one method to another

Consistency of a Dimensional Equation

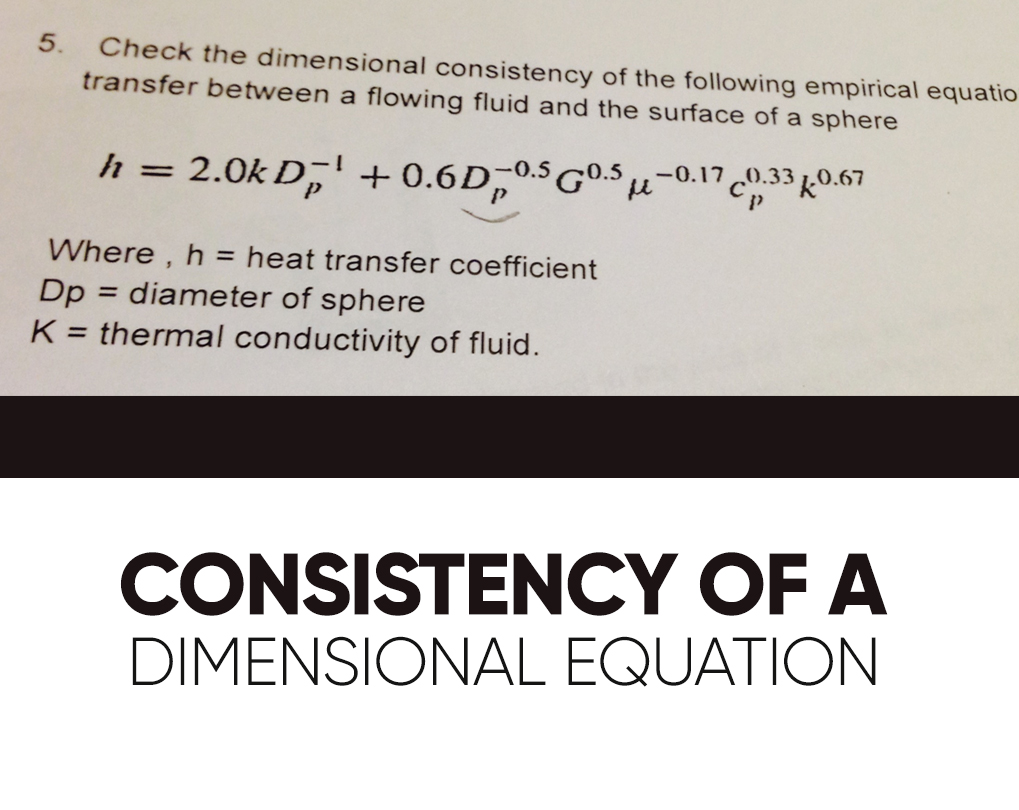
We can add or subtract physical quantities only if they have the same dimensions. The similarity of dimensions of physical quantities is called the consistency of a dimensional equation. As an instance consider velocity and mass, we cannot subtract or add these 2 physical quantities as they are of varied dimensions. That is [LT-1] for velocity and [M] for mass.

A dimension equation is said to be consistent, only and only if dimensions of equations are the same on both sides. If the dimensions of the equation are not the same on both sides then the equation is said to be dimensionally incorrect. Also, recognize that if an equation is dimensionally accurate it does not intend it is a perfectly correct equation.

Example:

Check the consistency of the dimensional equation of speed.

Speed = Distance/Time

[LT^-1] = L/T

[LT^-1] = [LT^-1]

The equation is dimensionally correct, as the dimension of speed is the same on both sides. Dimensional Analysis is a basic test to find out the consistency of the equation and doesn’t guarantee the correctness of the equation. One drawback of this method is that we can’t predict the constants of many physical quantities. Also, the logarithmic, trigonometric, and exponential function is dimensionless. For instance, take

The Angle of Sine = Length/Length is unit less, hence this is a dimensionless quantity.

Example 1

Check the consistency of the equation

x = x₀ + v₀t + (1/2) at²

Where x₀ and x are distances, v is velocity, t is time and a is an acceleration of the body.

Now to check if the above equation is dimensionally correct, we have to prove that dimensions of physical quantities are the same on both sides. Also, we have to keep in mind that quantities can only be added or subtracted if their dimensions are the same.

x = distance = [L]

x₀= distance = [L]

v₀t = [LT^-1] × [T] = velocity × time = [L]

at² = acceleration × time² = [LT^-2] × [T²] = [L]

Since dimensions of the left-hand side equal to dimension on the right-hand side, the equation is said to be consistent and dimensionally correct.

Example 2

Check whether the given equation is dimensionally correct.

W = 1/2 mv² – mgh

Where m indicates mass, v for velocity, g indicates gravity, W stands for work done, and h for height.

To verify the above equation as dimensionally right or wrong, we initial draft dimensions of all the physical quantities specified in the equation.

Work done (W) = [MLT^-2] × [L] (Force × Displacement) = [ML²T^-2]

Kinetic Energy= 1/2 mv² = [M] × [L²T^-2] = [ML²T^-2]

Potential Energy = mgh = [M] × [LT^-2] × [L] = [ML²T^-2]

Ago all the dimensions on the right and left sides are the same it is a dimensionally right equation.

FAQs

[wps_faq style=”classic” question=”Q: Why is dimensional analysis important?”]A: This method is one of the most famous and important mathematical tools in the subject of fluid mechanics. The main benefit of this method is that it decreases the number of variables in the problem by linking dimensional variables to form non-dimensional parameters.[/wps_faq][wps_faq style=”classic” question=”Q: Where is the dimensional analysis used?”]A: To verify the texture of a dimensional equation. To derive the connection between physical quantities in physical phenomena. To convert units from one system to another.[/wps_faq][wps_faq style=”classic” question=”Q: What are dimensional analysis limitations?”]A: This method cannot acquire a relationship or formula if a physical quantity depends upon more than 3 factors having dimensions. Dimensional analysis cannot derive a formula including exponential function, trigonometric function, and logarithmic function and it can’t acquire a connection having more than one part in an equation.[/wps_faq]

Conclusion

All the types of sciences and engineering make use of several units. Some of the obvious ones include length (yard, feet, inches, meters), mass (ton, ounces, pounds, grams, grains), and energy (Joule, erg, kcal, eV). Because there are so many various units that can be used, it is required to be strong to convert between the different units. To perform this, a conversion factor used.