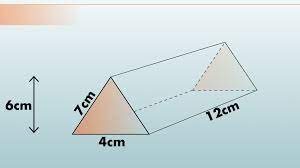
Calculating the surface area of a triangular prism may appear to be a bit complex at first glance, but fear not, for we have broken it down into simple steps for you.
Understanding the properties and formula is key, but the real challenge lies in…
Triangular Prism: Definition and Properties
A triangular prism is a polyhedron with two triangular bases and three rectangular faces. Imagine a shape resembling a Toblerone chocolate bar, with two triangular ends and three sides that are like the sides of a box. The bases are identical and parallel, while the rectangular faces connect the corresponding sides of the triangles. This type of prism is unique because of its combination of triangles and rectangles, giving it a distinctive appearance.
When you examine a triangular prism, you’ll notice that the two triangular bases are connected by three lateral edges, forming a prism that stands tall and sleek. The height of the prism is the perpendicular distance between the two bases. This height is crucial in calculating the surface area of the prism accurately. By understanding the basic properties of a triangular prism, such as its base, lateral edges, and height, you can begin to grasp how to navigate the calculations involved in finding its surface area.
Understanding Surface Area Formula
After familiarizing yourself with the properties of a triangular prism, calculating its surface area involves applying a specific formula based on its geometric characteristics.
The surface area of a triangular prism consists of the sum of the areas of its individual faces. To understand the formula better, let’s break it down.
For a triangular prism, the formula for surface area involves calculating the areas of two triangular bases and three rectangular faces. The formula can be expressed as SA = 2B + Ph, where SA represents the surface area, B is the area of one triangular base, P is the perimeter of the base triangle, and h is the height of the prism.
Step 1: Calculating Triangle Areas
To calculate the surface area of a triangular prism, you first need to determine the area of each individual triangle.
This involves using the triangle area formula, considering the base and height measurements of each triangle.
Triangle Area Formula
When calculating the surface area of a triangular prism, determining the areas of the individual triangles is a crucial first step. To find the area of a triangle, you can use the formula: Area = 0.5 * base * height. In this formula, the base represents the length of the triangle’s bottom side, while the height is the perpendicular distance from the base to the opposite vertex.
Remember, the base and height must be perpendicular to each other to accurately calculate the area. Once you have the measurements for the base and height of each triangle on the prism, plug them into the formula to find the area.
Calculating the areas of the triangles correctly is essential for determining the total surface area of the triangular prism accurately.
Base and Height
To calculate the area of a triangle, follow these steps:
- Identify the base and height of the triangle. The base is the bottom side, and the height is the perpendicular distance from the base to the opposite vertex.
- Ensure that both the base and height are measured in the same unit of measurement.
- If the base and height values are not provided, you can use the Pythagorean theorem to determine the height.
- Once you have the values for the base and height, plug them into the area formula: Area = 0.5 * base * height.
- Remember that the area of a triangle is always expressed in square units because it represents a two-dimensional space.
- Understanding the relationship between the base and height is essential for accurate calculation of the triangle’s area.
Finding Total Surface
To calculate the total surface area of a triangular prism, start by calculating the areas of the individual triangles that make up the prism. Each triangular face of the prism consists of a base and height. Use the formula for the area of a triangle, which is 0.5 times the base multiplied by the height.
Measure the base and height of each triangle, then multiply these values together and divide by 2 to find the area of each triangle. Once you have the areas of all the triangles, add them together to get the total surface area of the triangular prism.
This step is crucial in determining the overall surface area accurately and efficiently.
Step 2: Finding Prism’s Lateral Area
When calculating the surface area of a triangular prism, understanding how to find the prism’s lateral area is crucial.
You’ll need to consider the method used to calculate the lateral area, the perimeter of the triangle faces, and how it contributes to the prism’s total surface area.
This step is essential in accurately determining the overall surface area of the triangular prism.
Lateral Area Calculation Method
Calculate the lateral area of a triangular prism by finding the sum of the areas of its three lateral faces.
To do this, identify the base of the prism and its height.
The lateral faces of a triangular prism are all parallelograms with the base of the triangle as one side and the height of the prism as the other side.
Use the formula for the area of a parallelogram (base x height) to find the area of each lateral face.
Then, add these areas together to determine the total lateral area of the prism.
Remember, the lateral area doesn’t include the bases of the prism, only the three lateral faces.
This calculation is crucial for accurately determining the total surface area of the prism.
Triangle Faces Perimeter
Let’s now explore how to determine the perimeter of the triangular faces of the prism to calculate its lateral area effectively.
To find the perimeter of the triangular faces, you need to add the lengths of all three sides of the triangle. For each triangular face of the prism, identify the three side lengths and sum them up. This total will give you the perimeter of one triangular face.
Remember, the two triangular faces of the prism will have the same perimeter if the prism is regular.
Calculating the perimeter accurately is crucial for finding the prism’s lateral area, as it forms the base for further surface area calculations.
Understanding how to find the triangle faces’ perimeter is a key step in determining the prism’s total surface area.
Prism’s Total Surface
Wondering how to determine the lateral area of a prism efficiently? To find the prism’s total surface area, you first need to calculate the lateral area.
The lateral area of a prism is the sum of the areas of all the lateral faces. For a triangular prism, this means finding the area of all three rectangular faces. To do this, multiply the perimeter of the triangular base by the height of the prism.
This formula simplifies the process, ensuring you accurately compute the lateral area. By understanding and applying this method, you can efficiently determine the lateral area of a triangular prism, a crucial step towards calculating the prism’s total surface area.
Step 3: Determining Prism’s Base Area
To find the base area of the triangular prism, you need to determine the area of the triangle that forms the base. The formula for the area of a triangle is 1/2 multiplied by the base of the triangle multiplied by the height of the triangle. In the case of the triangular prism, the base is a triangle, so you’ll calculate the base area using this formula. Measure the base of the triangle and its corresponding height. Multiply these two values together, then divide the result by 2. This will give you the area of the triangle that forms the base of the prism.
Calculating the base area accurately is crucial because it serves as one of the components in finding the total surface area of the triangular prism. Once you have determined the base area, you can proceed to calculate the areas of the other faces of the prism to obtain the complete surface area. Stay focused on accurately measuring the base triangle to ensure the correctness of your calculations.
Step 4: Adding All Areas for Total Surface Area
To calculate the total surface area of the triangular prism, simply add together the areas of all its faces obtained in the previous steps.
Start by adding the area of the two triangular bases. If the base triangle has a base of length b and a height of h, the area can be calculated using the formula: 0.5 * b * h. Add this area for one base and then multiply by 2 to account for both bases.
Next, add the three rectangular faces’ areas. The area of a rectangle is determined by multiplying its length by its width. For a triangular prism, two of these faces will have a length equal to the base of the triangle and a height equal to the prism’s height, while the third face’s dimensions will be the prism’s height and the sum of the base’s sides.
Frequently Asked Questions
Yes, a triangular prism can have a right angle triangle as its base. This shape results in unique properties and angles, contributing to the prism’s overall structure and surface area calculation. It’s an interesting geometric configuration to explore.
When calculating surface area of a triangular prism, the orientation doesn’t impact the process. You still find the area of each face and add them up. Remember, all faces contribute to the total surface area, regardless of how the prism is positioned.
Yes, it is possible for a triangular prism to have equal side lengths for all its faces. This unique configuration results in an equilateral triangular prism, where all three sides of the base triangle and the lateral faces are of equal length.
Conclusion
You have now successfully calculated the surface area of a triangular prism by following the step-by-step guide provided.
By understanding the properties of a triangular prism and using the surface area formula, you were able to determine the total surface area by calculating the triangle areas, finding the prism’s lateral area, and determining the prism’s base area.
Keep practicing and applying these techniques to solve more geometry problems efficiently. Good job!