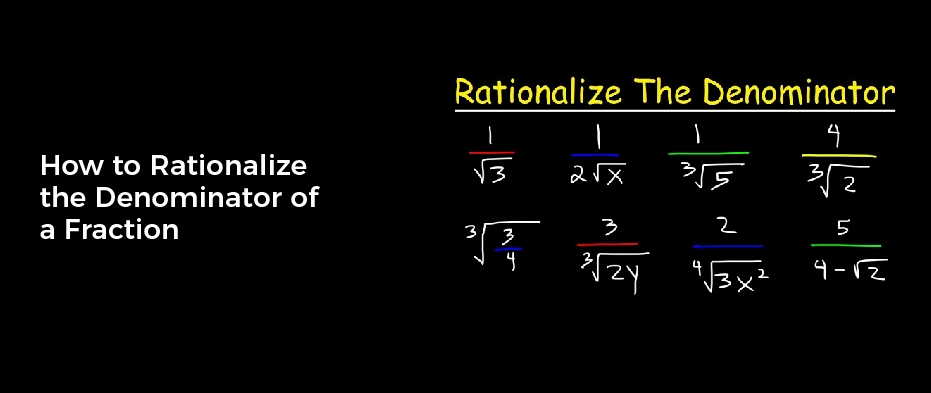
How to rationalize the denominator: As you can see, rationalizing the denominator can be a bit complex. However, with a few key steps and some practice, it is possible to master this concept and efficiently work through problems involving fractions. So if you are looking to improve your skills in rationalizing the denominator, start by familiarizing yourself with the basic steps and practicing as much as possible. With a little patience and persistence, you will be on your way to mastering this important concept in no time!
1. Multiply By the Radicand
When you multiply a radical and a quotient, it is a good idea to rationalize the denominator. This is a skill that can be useful in math class.
Rationalizing the denominator involves removing the radical from the denominator. It also involves finding the quantity that multiplies the denominator to create a rational number. The method is similar to that used to rationalize roots.
A common method for rationalizing the denominator is finding a factor with the same radicand as the original. You can use the Product Rule for this.
The Quotient Rule is another way to simplify a radical expression. Applying the Quotient Rule is a good idea if you have two or more terms in a fraction. That rule states that if a product is produced from a root and a quotient, the product is equal to the sum of the quotients.
Example
To rationalize a denominator, you need to multiply the denominator by a factor that has the same radicand and index as the original. In the example below, the numerator is 3. And the radical is XY.
If you have two or more different radicands in a fraction, combining them is a bad idea. You can only combine terms with the same radicand and index. Therefore, the best way to rationalize a denominator is to find the smallest factor with the same radicand.
2. Add Up Two Fractions with Irrational Denominators
If you are trying to add up 2 fractions with irrational denominators, then you will have to invert the denominator. Then, you can either multiply the numerator by the new numerator or add a new numerator.
Fractions are a form of numerical expression used primarily in schools and calculators. They can be represented as strings or with the float data type. You can then print them for easy textual representation.
When comparing fractions, you can use the common denominator. Common denominators are the same as the numerators of all fractions with the same denominator. This method is the best way to compare fractions.
3. Leave Transcendental Numbers in The Denominator
Transcendental numbers cannot be expressed as the roots of algebraic equations with rational coefficients. They are also the limits of infinite series. Many commonly used numbers are considered intangible. These include the square root of 3 and the cube root of 5.
In mathematics, a transcendental number is a type of real or complex number that is a limiting case of some sequence. It can be a ratio, a limit of an infinite series, or an expression of an endless continuing fraction.
The defining factor of a transcendental number is that it has a generating rule. This means that you can use any number to generate a transcendental number.
Example
If you have the square root of 3, the generating rule is that a factor of 2 multiplied by the square root of 3 will yield a transcendental number.
A more subtle way to find the generating rule for a number is to rationalize its denominator. This can be done by removing radicals from the denominator and finding a quantity that will multiply the denominator to make it rational.
While it is not as easy as replacing 5 with 2, rationalizing a denominator has become a standard “simplification” notion in basic mathematics. To rationalize the denominator of a binomial, you must first make sure the term is not multiplied by a radical.