When you find yourself in a laboratory setting, surrounded by various gases, the concept of partial pressure becomes crucial. Understanding how to calculate partial pressure using chemistry techniques can unlock a deeper comprehension of gas behavior and mixtures.
By mastering Dalton’s Law of Partial Pressure and grasping the significance of mole fraction in determining partial pressures, you’ll be equipped to navigate through real-world scenarios where these calculations are essential.
Dive into the intricacies of the Ideal Gas Law and explore practical applications that showcase the relevance of partial pressure in chemistry.
Gas Laws and Partial Pressure
When working with gases, understanding gas laws is crucial for calculating partial pressure accurately. Gas laws, such as Boyle’s Law, Charles’s Law, and Gay-Lussac’s Law, provide essential relationships between gas pressure, volume, temperature, and amount.
Boyle’s Law states that at constant temperature, the pressure of a gas is inversely proportional to its volume. Understanding this law helps you determine how changes in volume affect pressure and vice versa.
Similarly, Charles’s Law relates the volume and temperature of a gas at constant pressure. By grasping this law, you can predict how changes in temperature impact the volume of a gas.
Additionally, Gay-Lussac’s Law explains the relationship between gas pressure and temperature when volume is held constant. Mastering these gas laws equips you with the necessary tools to calculate partial pressure accurately in various scenarios.
Dalton’s Law of Partial Pressure
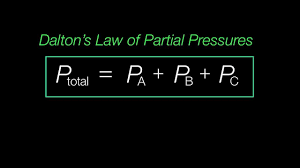
Understanding Dalton’s Law of Partial Pressure is essential for accurately determining the pressure exerted by each gas in a mixture. According to this law, the total pressure exerted by a mixture of gases is equal to the sum of the partial pressures of each individual gas in the mixture. This means that each gas in a mixture behaves independently of the others, exerting a pressure as if it were the only gas present in the container.
Dalton’s Law of Partial Pressure can be mathematically expressed as follows:
[ P_{ext{total}} = P_1 + P_2 + P_3 + … ]
Where ( P_{ext{total}} ) is the total pressure of the gas mixture, and ( P_1, P_2, P_3, ) and so on are the partial pressures of each gas component. This law is particularly useful when dealing with gas mixtures, such as those found in the atmosphere or industrial processes. By understanding Dalton’s Law, you can calculate the partial pressure of each gas in a mixture and predict how changes in conditions will affect the overall pressure.
Mole Fraction and Partial Pressure
To further explore the relationship between gas mixtures and their pressures, let’s now shift our focus to understanding the concept of mole fraction and its impact on partial pressure calculations. Mole fraction is a crucial aspect when dealing with gas mixtures. It’s defined as the ratio of the number of moles of a specific gas to the total number of moles in the mixture.
When calculating the partial pressure of a gas within a mixture, you can use the mole fraction to determine the proportion of that gas’s pressure contribution.
To find the partial pressure of a gas in a mixture, multiply the total pressure by the mole fraction of the gas in question. This calculation gives you the partial pressure exerted solely by that gas in the overall mixture.
Understanding mole fraction allows you to quantify the individual contributions of different gases to the total pressure in a system accurately. By utilizing mole fractions in partial pressure calculations, you can gain insights into the behavior of gas mixtures and make informed decisions in various chemical processes.
Ideal Gas Law Applications
Exploring the practical applications of the ideal gas law enhances your understanding of gas behavior in various scenarios. By utilizing the ideal gas law, which relates the pressure, volume, amount of substance, and temperature of a gas, you can solve a wide range of problems. For instance, you can determine the volume of a gas at a certain pressure and temperature or calculate the amount of gas present in a given volume. Additionally, the ideal gas law is crucial in studying gas mixtures, enabling you to find the partial pressure of each gas component within the mixture.
Furthermore, the ideal gas law is instrumental in various industries. For example, in the manufacturing sector, it’s used to optimize reaction conditions by controlling gas pressures and volumes. In environmental science, the ideal gas law helps in understanding the behavior of gases in the atmosphere. Whether you’re working in a laboratory setting or analyzing real-world phenomena, applying the ideal gas law provides valuable insights into gas properties and their interactions.
Real-World Examples and Practice
Enhancing your grasp of gas behavior through practical applications, real-world examples, and hands-on practice solidifies your understanding of the ideal gas law in action. By engaging in real-world examples, such as scuba diving, where partial pressures play a crucial role in understanding how gases behave under pressure, you can see firsthand how important these concepts are.
Imagine you’re diving deep underwater: as you descend, the pressure increases, causing the partial pressures of gases like oxygen and nitrogen to change. Through calculations based on the ideal gas law, you can determine these partial pressures and ensure your safety while exploring the depths.
Practicing with scenarios like this not only reinforces your knowledge but also prepares you for various situations where understanding partial pressures is vital. Whether it’s in scuba diving, industrial processes, or even studying the atmosphere, real-world examples help you apply theoretical concepts to practical settings.
Frequently Asked Questions
When at higher altitudes, the decrease in atmospheric pressure affects partial pressure calculations. Remember, the lower pressure leads to lower partial pressures of gases. Take this into account when determining the individual gas contributions in a mixture.
When calculating partial pressures and accounting for non-ideal behavior, adjust using the Van der Waals equation or other correction factors. Consider deviations from ideal gas assumptions like molecular volume and attractive forces to enhance accuracy in your calculations.
When using the ideal gas law to calculate partial pressures, keep in mind that it assumes ideal gas behavior, ignoring intermolecular forces and volume occupied by gas particles. This simplification may lead to inaccuracies in real-world scenarios.
Conclusion
So there you have it – calculating partial pressure in chemistry doesn’t have to be complicated. By understanding the gas laws, Dalton’s Law, and using mole fractions and the ideal gas law, you can easily determine the partial pressure of a gas in a mixture.
Remember to practice with real-world examples to solidify your understanding and application of these techniques. Keep practicing and you’ll be a pro at calculating partial pressure in no time!