Imagine expanded form as the blueprint that unveils the intricate structure of numbers, guiding you through their composition with clarity.
As you navigate through the layers of place value and decode the significance of each digit, a world of mathematical understanding awaits.
By unraveling the mystery of expanded form, you will gain a powerful tool to conquer complex numbers effortlessly.
Stay tuned to discover how this method not only simplifies calculations but also opens doors to real-world applications you never thought possible.
Understanding Expanded Form
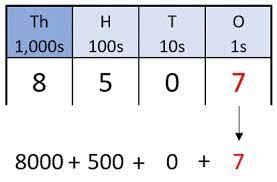
Understanding Expanded Form makes breaking down numbers into their component parts easier. Instead of seeing a number as a whole, Expanded Form allows you to see the value of each digit in relation to its place. For example, in the number 356, the 3 represents 300, the 5 represents 50, and the 6 represents 6. By breaking the number down this way, you gain a deeper understanding of its composition.
When you grasp Expanded Form, you can tackle larger numbers with confidence. Whether you’re dealing with three-digit numbers or even larger ones, the concept remains the same. Breaking down these numbers into expanded notation simplifies complex calculations and helps you visualize the magnitude of each digit’s contribution to the overall value.
Moreover, understanding Expanded Form sets a strong foundation for more advanced math concepts. It enhances your number sense and lays the groundwork for mastering topics like addition, subtraction, multiplication, and division. By mastering Expanded Form, you’re building a solid mathematical skill set that will serve you well in your academic journey.
Breaking Down Place Value
When it comes to understanding place value, remember that each digit holds a specific value based on its position in a number. Knowing the value of each digit is crucial for accurately representing numbers.
Take note of the importance of the position of digits as you break down the place value of a number.
Understanding Digits and Values
To grasp the concept of place value in mathematics, start by recognizing that each digit in a number holds a specific value based on its position.
In a multi-digit number like 354, the ‘3’ represents 300, the ‘5’ stands for 50, and the ‘4’ signifies 4.
The position of each digit determines its value – moving from right to left, the value of each digit increases tenfold. So, the ‘5’ in 354 is in the tens place, making it 50, while the ‘3’ in the hundreds place represents 300.
Understanding this relationship is crucial as it forms the foundation for working with numbers, making calculations, and interpreting numerical information accurately.
Importance of Position
Breaking down place value in mathematics reveals the significance of the position of each digit in a number. Understanding the importance of position is crucial in grasping the value of a digit within a number.
Each place in a number, whether it’s ones, tens, hundreds, or beyond, represents a different value. For instance, in the number 325, the ‘2’ holds a different value in the hundreds place than it would in the tens or ones place.
Recognizing the position of each digit helps in determining the overall value of the number. This concept is fundamental as it forms the basis for various mathematical operations, including addition, subtraction, multiplication, and division.
Emphasizing position in place value enhances your ability to work with numbers more effectively.
Converting Numbers Step by Step
Converting numbers step by step simplifies the process of breaking down large numbers into their individual place values. To begin, take a big number like 4,589. Start from the left and move to the right.
The first digit is in the thousands place, so the value is 4,000. The next digit is in the hundreds place, making the value 500. Moving on, the digit in the tens place holds a value of 80. Lastly, the digit in the ones place has a value of 9.
By breaking down the number into these place values, you can clearly see the components that make up the whole. This step-by-step method helps you understand the significance of each digit’s placement within the number. It also lays the foundation for grasping expanded form, where each digit is displayed with its respective place value.
Practice converting numbers in this manner to build a strong base for working with larger numbers in math.
Practice Makes Perfect
Enhancing your math skills through consistent practice is essential for mastering the concept of expanded form. By practicing regularly, you can develop a strong foundation and gradually increase your confidence in breaking down numbers into their expanded form. Start with small numbers and work your way up to more complex ones.
Set aside dedicated time each day to practice writing numbers in expanded form. Repetition is key to solidifying your understanding and improving your speed in converting numbers. Challenge yourself with different numbers and try to break them down in various ways to deepen your comprehension.
Don’t get discouraged if you find it challenging at first. Remember, practice makes perfect. The more you engage with expanded form, the easier it will become. Consider using online resources, worksheets, or even creating your own practice problems to enhance your skills.
Stay motivated and keep practicing, and soon you’ll find yourself breezing through numbers in expanded form effortlessly.
Mastering Decimals in Expanded Form
To excel in mastering decimals in expanded form, focus on applying the same foundational principles you’ve been honing with whole numbers. Decimals can seem tricky at first, but breaking them down into expanded form can make them much easier to work with. Remember, each digit’s place value is crucial in decimals, just like in whole numbers.
Start by understanding the value each digit holds based on its position after the decimal point. For instance, in the number 3.75, the 3 is in the ones place, the 7 is in the tenths place, and the 5 is in the hundredths place. To expand this decimal, you’d write it as 3 + 0.7 + 0.05.
Practice converting decimals to expanded form regularly to reinforce your understanding. As you become more comfortable with decimals, you’ll find that working with them in expanded form becomes second nature. Remember, practice makes perfect, and soon you’ll be a pro at mastering decimals in expanded form.
Strategies for Larger Numbers
When dealing with larger numbers, you’ll need to remember the importance of regrouping to simplify calculations.
Understanding the significance of place value is crucial for accurately representing numbers in expanded form.
Breaking down thousands into smaller, manageable parts can make working with big numbers less intimidating.
Regrouping for Big Numbers
When dealing with larger numbers, it becomes crucial to efficiently regroup in order to simplify calculations and avoid confusion.
Regrouping involves moving values between place values to make math operations easier. For instance, when adding or subtracting numbers with multiple digits, regrouping helps manage the carry-over or borrowing process.
In multiplication, regrouping allows you to break down the multiplication into smaller, more manageable steps. When dividing large numbers, regrouping helps in dealing with remainders and simplifying the process.
Place Value Importance
Understanding the significance of place value is essential for efficiently handling larger numbers in mathematics. When dealing with big numbers, each digit’s placement determines its value.
For instance, in the number 7,532, the ‘5’ holds a different value depending on whether it’s in the hundreds, tens, or ones place. Recognizing this helps you grasp the magnitude of each digit and how they contribute to the number as a whole.
By mastering place value, you can easily navigate through thousands, millions, or even billions without feeling overwhelmed. This knowledge forms the foundation for breaking down complex numbers into manageable parts, making arithmetic operations simpler and more straightforward.
Embrace the power of place value to conquer math challenges with confidence.
Breaking Down Thousands
To effectively tackle larger numbers, especially in the thousands range, employing strategic methods to break down these numerical behemoths is key.
When faced with a number like 5,678, start by identifying the digit in the thousands place, which in this case is 5. Understand that this digit represents 5,000.
Then, move on to the hundreds place, which is 6, representing 600.
Next, consider the tens place, which is 7, indicating 70.
Finally, look at the ones place, which is 8, representing 8.
Real-World Applications of Expanded Form
You can see the practical significance of expanded form when breaking down large numbers into their individual place values in real-life scenarios.
Imagine you’re at a store, and you need to calculate the total cost of 347 items priced at $25 each. Instead of multiplying 347 by 25 in one step, you can use expanded form to break down the multiplication into smaller, more manageable parts.
First, you can express 347 as 300 + 40 + 7, representing the hundreds, tens, and ones places respectively. Then, you multiply each part by 25 separately. For instance, 300 x 25 gives you 7500, 40 x 25 equals 1000, and 7 x 25 is 175. By adding these results together (7500 + 1000 + 175), you get the total cost of $8675.
This breakdown not only simplifies the calculation process but also helps you understand the value of each place in the final total. Real-world applications of expanded form like this can make complex math problems easier to tackle in everyday situations.
Frequently Asked Questions
Understanding expanded form aids problem-solving by breaking numbers into their components, enhancing your grasp of place value. It enables you to manipulate numbers efficiently, simplifying complex calculations and fostering a deeper understanding of mathematical concepts.
When breaking down place value with numbers in the millions or billions, you can easily identify the value of each digit by understanding expanded form. This helps in grasping the magnitude of large numbers and simplifying complex calculations.
When dealing with large numbers, look for patterns to speed up converting them into expanded form. Group digits based on their place value and tackle them in chunks. This method will help simplify the process.
Conclusion
You’ve got this! Remember, understanding expanded form is as simple as breaking numbers down into their place values.
With some practice and perseverance, you’ll be a pro at converting numbers into expanded form in no time.
Keep at it, and soon you’ll be able to tackle even the trickiest of math problems with ease.
Keep practicing and applying your knowledge to real-world situations, and you’ll see just how useful expanded form can be in everyday life.