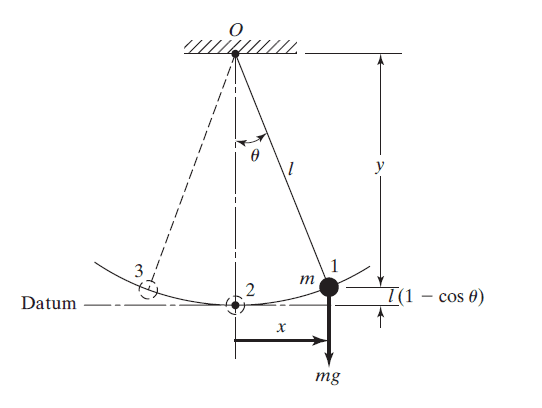
Did you know that finding the maximum acceleration is crucial in understanding the motion of objects? It’s a fundamental concept in physics that helps us analyze the speed and changes in velocity of an object.
But how exactly do you determine the maximum acceleration? In this discussion, we will explore the key factors that affect acceleration, the formulas used to calculate it, and real-world examples that demonstrate its relevance.
By the end, you’ll have a solid understanding of how to find maximum acceleration and its importance in the world of physics. So, let’s dive in and unravel the mysteries behind this intriguing concept.
Understanding Acceleration
To truly grasp the concept of acceleration, you must understand how it relates to velocity and time. Acceleration is the rate at which an object changes its velocity over time. In simpler terms, it measures how quickly an object speeds up, slows down, or changes direction. It’s important to note that velocity and acceleration aren’t the same. Velocity refers to the rate at which an object changes its position, while acceleration focuses on changes in velocity.
When an object accelerates, it means that its velocity is changing. If an object speeds up, its acceleration is positive. On the other hand, if an object slows down, its acceleration is negative. The magnitude of acceleration is determined by the amount of change in velocity and the time it takes for that change to occur.
Acceleration is a crucial concept in physics because it helps us understand how objects move and interact with their surroundings. It allows us to predict how fast an object can reach a certain velocity or how quickly it can change its direction. By studying acceleration, we can analyze the motion of objects and unravel the underlying principles that govern their behavior.
Definition of Maximum Acceleration
Now let’s talk about the points related to the definition of maximum acceleration.
Firstly, calculating maximum acceleration involves determining the change in velocity per unit of time. It can be calculated using the formula: maximum acceleration = (final velocity – initial velocity) / time.
Secondly, several factors can affect acceleration, such as the force applied, mass of the object, and any opposing forces like friction.
Calculating Maximum Acceleration
You can determine the maximum acceleration by calculating the rate at which an object’s velocity changes over time. To calculate maximum acceleration, you need to measure the change in velocity and the time it takes for that change to occur.
The formula for calculating acceleration is acceleration (a) equals the change in velocity (Δv) divided by the change in time (Δt). Δv is the final velocity minus the initial velocity, while Δt is the final time minus the initial time.
Factors Affecting Acceleration
Factors affecting acceleration can play a significant role in determining the maximum acceleration of an object. There are several key factors to consider.
- First, the mass of the object greatly impacts its acceleration. Heavier objects require more force to accelerate compared to lighter objects.
- Second, the force applied to the object is another crucial factor. The greater the force, the greater the acceleration.
- Third, the friction between the object and its surroundings can hinder acceleration. More friction means less acceleration.
- Additionally, the shape and aerodynamics of the object can affect acceleration. Objects with streamlined shapes experience less air resistance, allowing for greater acceleration.
- Lastly, external factors like gravity and other forces can also influence acceleration.
Understanding and manipulating these factors can help you determine and achieve the maximum acceleration of an object.
Key Factors Affecting Acceleration
What are the primary determinants of acceleration in physics?
When it comes to understanding acceleration, there are several key factors that play a crucial role in determining its value.
The first factor is the force applied to an object. According to Newton’s second law of motion, the acceleration of an object is directly proportional to the net force acting on it. In simple terms, the greater the force applied, the greater the acceleration.
Another factor is the mass of the object. The acceleration of an object is inversely proportional to its mass. This means that the smaller the mass, the greater the acceleration.
Additionally, the direction of the force is also important. Acceleration is a vector quantity, meaning it has both magnitude and direction. So, if the force applied is in the same direction as the object’s motion, the acceleration will be positive. Conversely, if the force is in the opposite direction, the acceleration will be negative.
Calculating Acceleration Using Formulas
To calculate acceleration using formulas, one must consider the key factors discussed in the previous subtopic. These factors include the change in velocity, the time taken for that change, and the initial velocity of the object.
The formula for acceleration is derived by dividing the change in velocity by the time taken. It can be expressed as a = (v – u) / t, where ‘a’ represents acceleration, ‘v’ represents final velocity, ‘u’ represents initial velocity, and ‘t’ represents time.
To use this formula, you need to know the initial and final velocities of the object and the time taken for the change in velocity to occur. By plugging in these values, you can easily calculate the acceleration.
It’s important to note that acceleration is a vector quantity, meaning it has both magnitude and direction. Therefore, you must also consider the direction of the velocity change when calculating acceleration.
With the proper values and the formula at hand, you can calculate acceleration and gain a deeper understanding of an object’s motion.
Finding Maximum Acceleration in Uniform Motion
Now let’s talk about the points to consider when finding maximum acceleration in uniform motion.
First, you need to understand how to calculate acceleration values using the appropriate formulas.
Next, it’s important to be aware of the factors that can affect acceleration, such as mass and force.
Lastly, a graphical representation of acceleration can provide a visual understanding of how it changes over time.
Calculating Acceleration Values
To calculate the maximum acceleration in uniform motion, start by determining the rate of change in velocity over time. This can be done by finding the difference between the final velocity and the initial velocity, and then dividing that difference by the time taken.
The formula for acceleration is:
Acceleration = (Final Velocity – Initial Velocity) / Time
For example, let’s say you’re driving a car and you want to find its maximum acceleration. You measure the initial velocity as 20 meters per second and the final velocity as 40 meters per second. The time taken to achieve this change in velocity is 5 seconds.
Plugging these values into the formula, you get:
Acceleration = (40 m/s – 20 m/s) / 5 s
Acceleration = 20 m/s^2
Therefore, the maximum acceleration of the car is 20 meters per second squared.
Factors Affecting Acceleration
Factors affecting acceleration in uniform motion can include the mass of an object and the amount of force applied to it.
The mass of an object plays a significant role in determining its acceleration. According to Newton’s second law of motion, the acceleration of an object is directly proportional to the force applied to it and inversely proportional to its mass.
In simpler terms, a smaller mass will experience a greater acceleration for the same amount of force applied. On the other hand, a larger mass will require a greater force to achieve the same acceleration.
Therefore, when trying to find the maximum acceleration in uniform motion, it’s essential to consider the mass of the object and the force applied to it.
Graphical Representation of Acceleration
When analyzing the maximum acceleration in uniform motion, it’s crucial to understand its graphical representation. By plotting the acceleration values on a graph, you can easily determine the maximum acceleration.
Start by labeling the x-axis as time and the y-axis as acceleration. Mark the data points for each time interval and corresponding acceleration value. Connect these points with a straight line. The steeper the line, the greater the acceleration.
To find the maximum acceleration, look for the highest point on the graph, which represents the peak of the acceleration values. By visually analyzing the graph, you can quickly identify the maximum acceleration in uniform motion.
This graphical representation provides a clear and concise way to determine the maximum acceleration.
Finding Maximum Acceleration in Non-Uniform Motion
You can determine the maximum acceleration in non-uniform motion by analyzing the rate of change of velocity over time. In non-uniform motion, the velocity of an object changes at varying rates. To find the maximum acceleration, you need to examine the intervals where the rate of change of velocity is the greatest.
This can be done by calculating the derivative of the velocity-time graph or by analyzing the instantaneous acceleration at different points in time.
One way to find the maximum acceleration in non-uniform motion is by calculating the derivative of the velocity-time graph. The derivative represents the rate of change of velocity with respect to time. By finding the points on the graph where the derivative is the highest, you can determine the intervals with the maximum acceleration.
Another method is to analyze the instantaneous acceleration at different points in time. Instantaneous acceleration is the acceleration of an object at a specific moment in time. By calculating the difference in velocity over a small time interval and dividing it by that interval, you can find the instantaneous acceleration. By analyzing the values of instantaneous acceleration at different points in time, you can identify the moments with the maximum acceleration.
Real-World Examples of Maximum Acceleration
Real-world examples demonstrate the application and significance of maximum acceleration in various contexts.
One such example is the world of sports, where athletes strive to achieve maximum acceleration in order to gain a competitive edge. Take sprinters, for example. When the starting gun goes off, they aim to reach their maximum acceleration as quickly as possible to cover the shortest distance in the shortest amount of time.
Similarly, in motorsports, drivers push the limits of their vehicles to achieve maximum acceleration on straightaways, allowing them to overtake opponents and gain an advantage.
Another example can be found in roller coasters. These thrilling rides are designed to provide passengers with an exhilarating experience by subjecting them to high levels of acceleration. When a roller coaster rapidly ascends a steep hill or enters a loop, it experiences maximum acceleration, giving riders a sense of weightlessness and excitement.
In the field of space exploration, rockets rely on maximum acceleration to overcome Earth’s gravity and reach escape velocity. The immense power generated by rocket engines allows them to achieve high acceleration, propelling spacecraft into orbit and beyond.
Tips for Applying Maximum Acceleration in Physics Problems
To effectively apply maximum acceleration in physics problems, it’s essential to understand the fundamental principles and equations that govern this concept. One tip for solving these problems is to identify the given information and what needs to be solved. This will help you determine which equations to use and how to apply them.
It’s also important to understand the relationship between acceleration, velocity, and time. In many cases, you may need to use the kinematic equations, such as the equation that relates final velocity, initial velocity, acceleration, and time.
Another tip is to pay attention to the units of measurement. Make sure all the quantities you’re working with have the same units to ensure accurate calculations.
Additionally, it’s helpful to break down the problem into smaller steps. By tackling one part of the problem at a time, you can avoid confusion and potential errors.
Frequently Asked Questions
How Does Maximum Acceleration Differ From Average Acceleration?
Maximum acceleration differs from average acceleration because it measures the highest rate of change in velocity at any given time, while average acceleration calculates the average rate of change in velocity over a period of time.
What Are Some Common Units Used to Measure Acceleration?
To measure acceleration, you commonly use units like meters per second squared (m/s^2) or feet per second squared (ft/s^2). These units represent the change in velocity over time, helping you determine the rate of acceleration.
Can Maximum Acceleration Be Negative?
Yes, maximum acceleration can be negative. It represents a change in velocity in the opposite direction. Negative acceleration is commonly seen when an object is slowing down or moving in the opposite direction.
Is It Possible for an Object to Have a Maximum Acceleration of Zero?
Yes, it is possible for an object to have a maximum acceleration of zero. This means that the object is not accelerating at its maximum potential and is maintaining a constant velocity.
How Does Air Resistance Affect Maximum Acceleration?
Air resistance can affect maximum acceleration by opposing the motion of an object. The greater the air resistance, the more it slows down the object, reducing the maximum acceleration that it can achieve.
Conclusion
In conclusion, understanding maximum acceleration is crucial in the field of physics. By calculating acceleration using formulas and considering key factors, we can determine the maximum acceleration in both uniform and non-uniform motion.
Real-world examples provide practical applications of this concept. By applying the knowledge of maximum acceleration in physics problems, we can solve various scenarios and gain a better understanding of the laws of motion.