Analyzing Rational Functions: How to Find Holes in the Graph

Discover how to uncover hidden gaps in graphs of rational functions with our step-by-step guide. Unleash your analytical skills now!

Are you tired of tirelessly graphing rational functions, only to be left with unanswered questions?

Well, fear not, because in this discussion, we will unravel the mystery of finding holes in the graph of rational functions.

By analyzing the characteristics of these elusive holes, we will equip you with the techniques needed to uncover them.

So, get ready to take your understanding of rational functions to the next level as we delve into the world of holes in the graph.

Understanding Rational Functions

To understand rational functions, you need to grasp the relationship between polynomials and the division of two polynomials. Rational functions are functions that can be expressed as the quotient of two polynomials. The numerator and denominator of a rational function are both polynomials, and the degree of the denominator can’t be greater than the degree of the numerator.

The relationship between polynomials and the division of two polynomials is crucial in understanding rational functions. Division of two polynomials involves dividing the coefficients of the terms in the numerator by the corresponding terms in the denominator. This process helps simplify the rational function and allows us to analyze its behavior.

When working with rational functions, it’s important to identify any restrictions on the domain. These restrictions occur when the denominator of the rational function is equal to zero. The values that make the denominator zero are called the vertical asymptotes of the function. They represent points where the function is undefined.

Graphing Rational Functions

Now, let’s explore how to graph rational functions by analyzing their key features and using techniques such as finding intercepts and asymptotes.

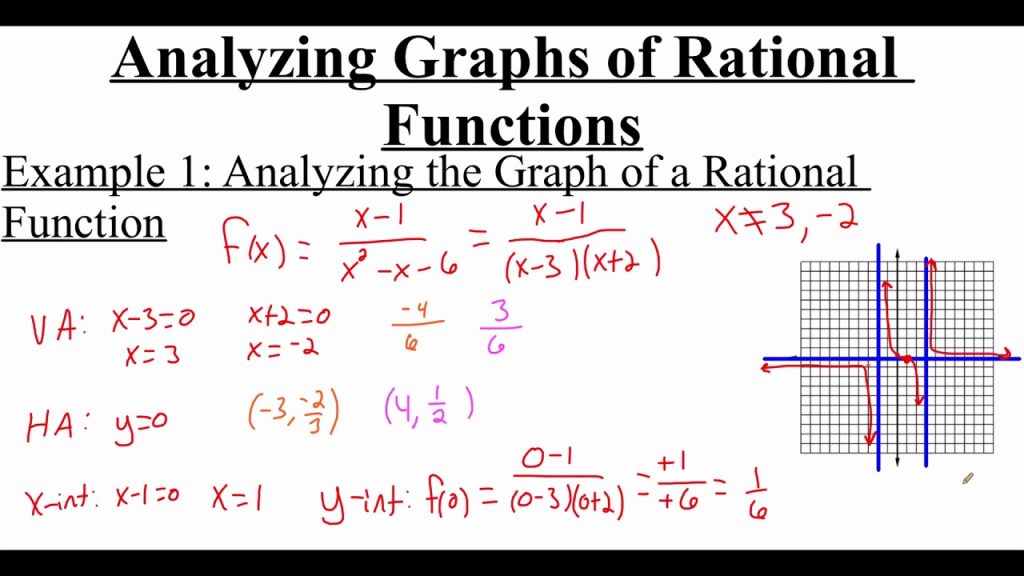
When graphing a rational function, it’s important to first identify any vertical asymptotes. These are values of x that make the denominator of the function equal to zero. To find these asymptotes, set the denominator equal to zero and solve for x. Once you have identified the vertical asymptotes, plot them on the graph as vertical dotted lines.

Next, find the x-intercepts by setting the numerator equal to zero and solving for x. These are the values of x where the graph crosses the x-axis. Plot these points on the graph as solid dots. Additionally, find the y-intercept by evaluating the function at x = 0. This is the point where the graph crosses the y-axis.

After finding the intercepts and asymptotes, you can plot additional points to get a better sense of the graph’s shape. Choose values of x on either side of the asymptotes and evaluate the function to find the corresponding y-values. Connect these points smoothly to create the graph.

Remember to label the intercepts, asymptotes, and any other important points on the graph. Graphing rational functions can be challenging, but by following these steps and paying attention to the key features, you can accurately depict the function on a graph.

Identifying Holes in the Graph

Now let’s talk about identifying holes in the graph of rational functions.

A hole occurs when a value in the function’s domain causes both the numerator and denominator to equal zero, resulting in an undefined point on the graph.

To identify holes, you can simplify the rational function and cancel out common factors between the numerator and denominator.

Hole Definition and Examples

Identify holes in the graph of a rational function by locating points where the function is undefined. A hole in the graph occurs when there’s a common factor between the numerator and the denominator of the rational function that cancels out at a certain point.

This causes the function to be undefined at that specific point. To find the points where the function is undefined, set the denominator equal to zero and solve for x. These values of x represent the x-coordinates of the holes in the graph.

For example, if the denominator of a rational function is (x – 2)(x + 3), then the function is undefined at x = 2 and x = -3. These points will be the location of the holes in the graph.

Techniques for Hole Identification

To identify holes in the graph of a rational function, examine the points where the function is undefined by setting the denominator equal to zero and solving for x. These points are called potential holes because they may or may not exist on the graph.

Once you have found the x-values that make the denominator zero, you can simplify the function by canceling out any common factors between the numerator and denominator. This will help you determine if the potential hole is an actual hole.

If after simplifying the function, the common factors cancel out, leaving a hole at the x-value you found earlier, then you have successfully identified a hole in the graph.

Remember to always check for holes in rational functions as they can provide valuable information about the behavior of the function.

Characteristics of Holes in Rational Functions

Understanding the characteristics of holes in rational functions is essential for analyzing their behavior and making informed mathematical decisions. Holes in rational functions occur when both the numerator and denominator of the function have common factors that can be canceled out, resulting in a hole in the graph.

One characteristic of holes is that they’re always removable discontinuities. This means that although there’s a hole in the graph, the function can still be defined at that point by removing the common factors.

Another characteristic of holes is that they appear as a single point on the graph. Unlike vertical asymptotes, which extend indefinitely, holes are finite points on the graph where the function is undefined but can be made continuous by canceling out the common factors.

Holes can also be identified by finding the x-values where the function is undefined and determining if the factors in the numerator and denominator can be canceled out. If they can, then there’s a hole at that x-value.

Understanding these characteristics of holes in rational functions is crucial for accurately analyzing the behavior of the function and making informed decisions about its graph. By identifying and understanding holes, you can gain a deeper understanding of the function’s behavior and make more accurate mathematical predictions.

Techniques for Finding Holes in the Graph

To find holes in the graph of a rational function, you can investigate common factors in the numerator and denominator. This technique involves looking for values of x where both the numerator and denominator are equal to zero. These common factors can cause the function to be undefined at certain points, creating holes in the graph.

To start, factor both the numerator and denominator completely. Look for any factors that are common to both expressions. These common factors represent potential holes in the graph. Set each common factor equal to zero and solve for x. The values of x that make these common factors equal to zero are the x-coordinates of the holes in the graph.

Once you have identified the x-coordinates of the holes, substitute these values back into the original rational function to find the y-coordinates. These y-coordinates will complete the coordinates of the holes.

It is important to note that not all rational functions will have holes in their graphs. Some may have vertical asymptotes or horizontal asymptotes instead. However, if there are common factors in both the numerator and denominator, there’s a high likelihood of finding holes in the graph. By investigating these common factors, you can accurately identify and analyze the holes in the graph of a rational function.

Interpreting Holes in Rational Functions

When interpreting holes in rational functions, it’s important to analyze the impact of these undefined points on the overall behavior and characteristics of the graph. A hole occurs in a rational function when both the numerator and denominator of the function have a common factor that cancels out. This results in an undefined point where the function is discontinuous.

Holes in rational functions have a significant influence on the graph’s behavior. They create gaps or breaks in the graph, causing the function to have distinct sections. The presence of a hole indicates that the function isn’t defined at that particular x-value. Therefore, it’s crucial to identify these points and understand their implications.

Furthermore, holes affect the asymptotic behavior of the graph. As the function approaches the x-value of the hole, it approaches a different y-value from both sides. This creates a discontinuity in the graph, causing it to have different limits at that x-value.

Frequently Asked Questions

Can Rational Functions Have More Than One Hole in Their Graph?

Yes, rational functions can have more than one hole in their graph. The holes occur when there are common factors in the numerator and denominator that cancel out, resulting in a discontinuity.

Is It Possible for a Rational Function to Have a Hole at the Vertical Asymptote?

Yes, it is possible for a rational function to have a hole at the vertical asymptote. This occurs when there is a common factor between the numerator and denominator that cancels out.

What Is the Significance of the X-Value at Which a Hole Occurs in a Rational Function?

The x-value at which a hole occurs in a rational function is significant because it represents a point where the function is undefined. It is important to identify and understand these holes in order to accurately analyze the graph.

Are There Any Specific Patterns or Characteristics That Can Help Identify the Presence of a Hole in a Rational Function’s Graph?

To find holes in a rational function’s graph, look for values of x that make the denominator zero but cancel out in the numerator. These locations will exhibit a hole where the function is undefined.

Can the Presence of a Hole Affect the Domain and Range of a Rational Function?

Yes, the presence of a hole can affect the domain and range of a rational function. It can cause restrictions on certain x-values and limit the possible y-values on the graph.

Conclusion

In conclusion, understanding and graphing rational functions can be a complex task.

However, by learning how to identify and analyze holes in the graph, we gain valuable insights into the behavior of these functions.

By using techniques such as factoring and simplifying, we can uncover the location and characteristics of these holes.

Interpreting these holes allows us to understand discontinuities and restrictions in the function, leading to a deeper understanding of rational functions.