What Does Domain and Range Mean in Math

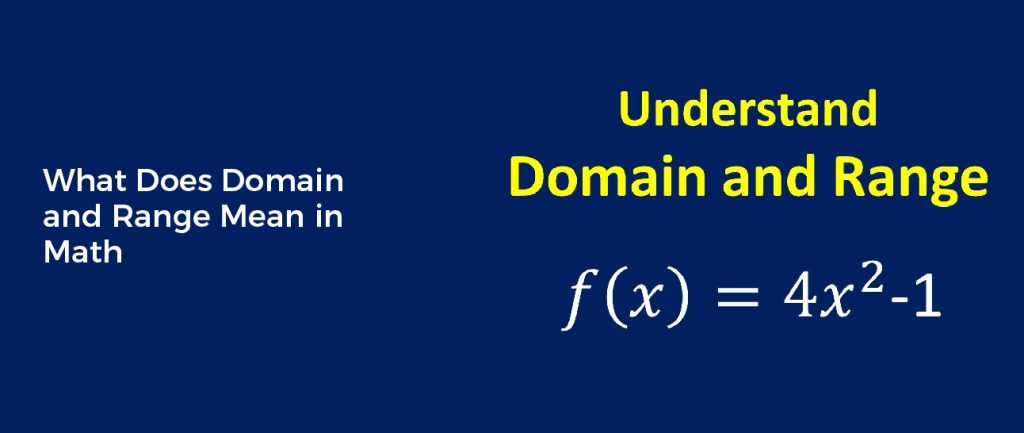
Do you ever get confused when math terms like domain and range come up in discussions? You’re not alone! Many students feel overwhelmed by these mathematical concepts. In simple terms, the codomain and range are used to describe a set of values that allow us to draw conclusions about equations. Whether you’re struggling with basic algebra or understanding higher-level calculus equations, the concept of codomain and range will always be important for understanding data sets within mathematics.

Through this blog post, we explore what exactly is meant by ‘domain’ and ‘range’ and how to identify them in equations – demystifying one of mathematics’ most confounding topics!

The Basic Terms

Co-domaindescribes what inputs are valid for the function, while the range describes the outputs of the function. For example, if a function has an equation y = x2 + 5, then its domain would be all real numbers, that is, 5.

Ordered pairsare a type of mathematical relationship. They can be shown in various ways. Graphs are often used to show this, but a table can also represent it. Typically, a table will have a domain on the left and a range on the right. The domain is a set of all possible input values. Similarly, the range is a set of all possible output values.

Disjoint intervalsare formed when overlapping intervals are combined. These intervals are sometimes called ranges and domains. They can be used to describe functions.

What Is Domain?

Domain and range are two important concepts in mathematics, and these two factors determine the codomain and range of a function. The first is the relationship between the dependent variable and the independent variable. It may be limited to a certain number of discrete values, or it may be infinite. Usually, a domain of a function is a set of all positive real numbers.

They are used to describe the set of possible outputs and inputs for a function. These can be found by graphing the function or by using a table. A domain and range calculator can also be helpful when checking your work.

How to Find the Domain of a function?

• The domain will be determined by looking at the values of the independent variables, which should not contain a zero at the bottom and a negative sign inside the square root.
• Domains of functions are restricted to all sets of real numbers (R). These are:
• When the given function is of form f(x) = 2x + 5 or f(x) = x2 – 2, the domain will be “the set of all real numbers.
• When the given function is f(x) = 1/(x – 1), the domain will be the set of all real numbers except 1.
• Sometimes, the interval is specified along with the function, such as f(x) = 3x + 4, 2 < x < 12. Here, x can take the values between 2 and 12 as input (i.e., domain).
• Domain restrictions refer to the values that are not defined by the function.

What is Range?

Domain and range are two basic mathematical concepts. They describe the set of values that a function takes. In addition to being used to show mathematical relationships, they are also used to create a visual representation of functions.

The domain of a function is a set of all possible inputs and outputs that are x-values and y-values. It can be used to illustrate the function in a graph or an equation. A simple linear function is a good example of demonstrating domain and range. For instance, the domain of the function f is -5 to the right, and f(x)=4x+3 represents a set of positive real numbers.

The range of a function is a list of all possible outputs. It can be used to demonstrate the function in a graph or an interval notation. Generally, the range will include all values in the second column. However, it can be restricted to a certain number of discrete values.

How to Find the Range of a function?

Here is the consider a function y = f(x).

• The spread of all the “Y” values from min to max is the range of the function.
• In the given expression of y, substitute all the values of x to check whether it is positive, negative, or equal to other values.
• You can find the min and max values for y.
• Then you have to draw a graph for the same.